Moving to a new blog site

I hope you will join me at my new blog site, Striving Learners. It’s where I write about supporting student learning as a 6-8 math interventionist in the classroom, in homeroom support, and in our district’s Title I after school program.

This blog has served me well, but I wanted to create a new space to write with a sharper focus on striving learners experiencing the Illustrative Math/Open Up resources curriculum.

Please explore my new site. It contains links to many documents which you can use to preview and reteach the 6-8 IM curriculum.

Success criteria and Open Up resources

Of late, I have been on thinking a LOT about criteria for success thanks to my colleague Cathy Dickson. She came upon an Open Up Facebook post and noticed that teachers have different interpretations of success criteria.

Our understanding of success criteria is different than what the two teachers describe. Since our district offers both Skillful Teacher and more recently High Impact Teacher Teams, through Research for Better Teaching, this is where my understanding is rooted. Anyway, in order to discuss success criteria we need to have a common understanding. For me it is either an oral or written description of what success looks like. Students refer to the criteria when completing a task and refer to it again for reflection. This post focuses on providing written success criteria.

Having a criteria for success (CfS) is becoming more and more apparent to me, and we are beginning to discuss them in our PLC’s. For example, after an assessment last week one of the 8th grade teachers asked students to reflect on their performance. The question was open ended and later we were wondering if a CfS would have provided focus.

Writing CfS isn’t as easy as it seems–especially when your resource is problem based. What attributes should be included? Have I accounted for multiple ways of problem solving? When should the criteria include a checklist for procedural fluency and when should it be written for conceptual understanding?

Technically every lesson should have success criteria, but who has time for that. If you read this blog you know we are piloting the Open Up resource. Writing CfS hasn’t been on my radar but I needs to be. When we ask students to perform a task then reflect on their learning, either through a cool down or formative assessment, they need to know the criteria for success.

I wanted some practice writing CfS so I spent some time drafting one for this 8th grade Unit 4 Lesson 13 cooldown only to have another colleague suggest the cooldown may not meet the lesson objective. The actual learning targets for the lesson are:

• I can graph a system of equations.
• I can solve systems of equations using algebra.

If you’ve already taught this lesson let me know your thoughts. Anyway, after going through the lesson I am wondering if these learning targets might be a better match for the cooldown:

• I can identify the number of solutions to a system from a graph.
• I can write a system of equations from a graph.

Here’s the cooldown:

Since no intervals are provided the response it is a bit more open ended so the criteria needs to account for that. Below is a draft. The green column indicates what the student would initially be provided on the first look. In yellow is the feedback the student would receive for self assessment purposes. Afterwards students would turn it in to the teacher or place them in bins similar to what Morgan Stipe does in her classroom.

This cooldown lends itself to providing feedback that, I think, requires little explanation so students can simply read the yellow portion to determine which feedback applies to them. This is a work in progress so I’m sure I’ve missed something. Let me know.

Also, if you’ve created CfS for or with your students I would love to learn more about it.

Middle school SMART goal setting

Yesterday on Twitter, math teacher Sarah Martin and I briefly commiserated on student behavior and how it impacts learning. Coincidently I had the same discussion with our 8th grade math teachers the previous week. We talked about a range of behaviors–students talking out of turn, or not participating, others not taking ownership of their learning and being off task. We created this list with specific students in mind.

Establishing SMART Goals

Our first meeting led to a second. This time we invited our social work intern to get her insight on establishing and monitoring goals. We shared a few ideas we had in mind for particular students, but she was quick to point out that it is essential for the student to have input to create buy-in. I’m not sure why that fell off my radar, but it did. As we talked we realized every student, not just the striving ones, could benefit from a SMART goal.

A few students might create their own, but we identified six goals that will fit most of our students’ needs:

• I will raise my hand to participate to in class. This goal is for students who continually blurt out, or students who do not participate.
• I will use whole body participation by: maintaining eye contact, keeping mouth quiet, keeping body still, facing the speaker, and respecting the speaker. This goal is for students who are off task in either whole class or small group work.
• I will seek timely support from a teacher when I don’t understand or to confirm I understand a concept. I will do this by asking a question in class, or homeroom. This goal is for students who delay seeking or do not seek support.
• I will complete at least 60% of assignments each week (e.g., 3 out of 5, 3 out of 4, 2 out of 3). This goal is for students who struggle with homework completion.
• I will lead my group in small group tasks. This goal is for students who are reluctant lead learners–those who lack confidence, or those who prefer to work alone.
• I will organize my binder. This goal is for students who need to declutter their binder.

Monitoring SMART Goals

We ended the meeting, without resolution, on identifying an efficient means to monitor every student’s goal without adding too much to our plate. We joked about goal setting apps for students, so after the meeting I did a quick search. Unfortunately, I couldn’t find any apps where the goals could be shared and monitored by the teacher.

A few days passed when I realized this Google Sheets file that I had been toying with for a different purpose could be put to use. Below is a gif which simply shows how the student would only need to type in a number 1-4 to capture progress.

The plan is to push out to students variations of this file using Canvas, where students would make a copy and rename their file. Each week during class students will report their progress.

Instead of the teacher opening 70 plus google sheets to monitor progress, students would take a screenshot of their progress and submit it to Canvas. That would likely be a more efficient method for the teacher to provide feedback.

Recognizing student progress

The last piece to this puzzle is student recognition. I’m thinking every 2-3 weeks students are briefly recognized for their progress. Depending on the student’s request the recognition could be a personal mention, or a more formal class announcement. I still need to flesh this out.

I know this plan will not change every student’s behavior overnight. However a few minutes each week pondering your goal may be just what some students need.

Adding tape diagrams to IM’s card sorts allow greater access to striving students

I’ve neglected to blog for a bit because I have been consumed with creating Illustrative Math resources that support student learning in our Title I after school math program.

It has been quite a challenge adapting and modifying our old resource to match IM’s deep conceptual understanding and lesson progression. Revising after school lessons that model and reinforce IM’s approach to learning isn’t easy. In many cases I’ve had to start from scratch.

Two activities worth sharing are modifications I made to seventh grade Unit 4 Proportional Relationships and Percentages.

Students in the after school program benefit from visual models so I wanted to add tape diagrams to the Unit 4 Lessons 4 and 5 card sorts. Since the 7th grade teachers were using IM’s card sorts in class, I needed to created new situations, equations, and tables along with the tape diagrams. I combined IM’s two card sorts since the students already received instruction on Lesson 5.

Below is an example of IM’s card sort.

Here’s my modification.

Adding the tape diagrams really allowed our striving students to better connect the situation with the equation and table.

The strategy was so successful I used it to reteach IM’s Lesson 6 Increasing and Decreasing. Students were given this handout (key included) and collaborated on completing the tape diagram, finding the percent increase or decrease, and creating the equation.

 

Feel free to use these two activities and let me know if they are helpful.

 

How much should study guides mimic an assessment?

Last week during parent-teacher conferences an 8th grade parent stated their student saw the pattern between the study guide problems and the assessments. Mom didn’t say whether it was good or bad, it was just a statement of fact. Obviously we don’t want students to be surprised on an assessment, but are our study guides overly transparent?

This has been on my mind since Bryan Meyer tweeted images from @ilana_horn‘s book Motivated. Most of our grade 6-8 math study guides mirror problems that are on the assessment. Are we distorting grades by doing so? Could we include a few study guide problems as opportunities to deepen learning while “protecting the integrity” of the assessment? What would that look like?

When studying 6th grade ratios, the overarching goal of the standard is for students to use ratio reasoning to solve problems. For example if students use a double number line to solve for a missing quantity our typical study guide would replicate that problem using different numbers and ingredients. Instead of mirroring the problem, what if the study guide showed a double number line and the students were asked to pose a question then answer it? Would that develop deeper learning?

I tried this idea on two students during math support. At first they were stumped. It never occurred to them to think this way. They were used to “problem solving”, not “problem posing”. Once they understood what I was asking they were able to pose a question, but their questions lacked clarity. Then, as you can see from the photo, their questions were much more clear. As an aside, every question they posed, while accurate, resulted in an answer that was found in the second part of double number line.

A problem posing question is not on our assessment, however I could see this type of problem being valuable on a study guide as long as students have had some practice posing questions.

In seventh grade the end of unit assessment is on proportionality. One problem on the assessment asks students to examine an off the grid line graph where they are to determine all true answers about a proportional relationship when given a point on the line. Another problem from the assessment asks students to examine a different off the grid line graph with a point whose coordinates represents the unit rate.  They are then asked to write the equation for the proportional relationship.

My idea for the study guide is to pose this problem–something I modified from Khan Academy. It’s still a work in progress.

Writing the equation for this relationship may be challenging since the constant of proportionality isn’t identified by the ordered pair of (1, 40)  But there are enough clues for students create a table. Doing so might indirectly address identifying  “true” statements about the relationships.

I would love to hear others’ thoughts on study guides. The conversation I am having in my head is also spurred by the piloting of the Illustrative Math resource. Study guides are currently not a part of this resource. If IM is in the process of creating study guides, I wonder if the problems mirror the assessment, or are they different?

 

 

Clarifying the learning journey; revising IM’s learning targets

 

As we continue our Illustrative Math pilot and prepare for the next unit, the sixth grade teachers recently examined the learning targets identified in Grade 6, Unit 2, Introducing Ratios. We took a deep dive using a document created by @normabgordon  in which she captured all the (@openupresources ) IM learning targets in Unit 2.

We appreciate IM’s work, but to clarify our students’ learning journey, we determined many targets needed revision. Some edits were minor, others required more tweaking. Here is our interpretation of Grade 6, Unit 2. Below is a selection of revised targets based on our district’s brief list of “look fors”.

Using student friendly language

The IM targets were student friendly, however we wanted to make them more concise. For example in Lesson 2:

…and in Lesson 9:

We felt these changes still capture the essentials without jeopardizing the learning intentions.

Selecting “observable” verbs to describe Bloom’s Taxonomy or Depth of Knowledge level

IM did a good job of selecting “observable” verbs for the learning targets, however the verb “know” was used in six of the 17 lessons in the unit. Since it’s difficult to observe “know”, we chose different verbs that are observable–such as identify, or explain. For example in Lesson 11:

Note: we also combined the second and third learning targets and made the targets more brief.

 

“Understand” is also unobservable so we revised this learning target as well.

Omitting context

There’s some debate on whether to include the context in which the outcome will be observed. When creating learning targets, we’ve been advised to omit the context as it relates to the learning activity. For example in Lesson 4 the targets included  color mixtures which we omitted:

I appreciate the heavy lifting IM has done in writing learning targets for each lesson. It is always easier to revise than to start from scratch. But the other sixth grade teachers and I felt it necessary to clarify the learning journey a bit more. Tackling the targets was not a solo effort. I work with a great group of teachers both in the building and at our “sister” middle school and they deserve a shoutout: @CathyDicksonD95, @MichelleRuotolo ,  @Mr__Manahan, and  @MrsOlsenLZ.

 

 

 

 

 

 

 

Friends of the Library Book Sale task

The other day a friend of mine, who is a Friends of the Library board member, presented me this real life dilemma which might make for an interesting  group task.

Hey, Mary!

We’re getting ready for our Book Sale and we’ll be unpacking hardcover books stored in Hinckley & Schmidt water boxes. We want to display the books spine up on 8ft x 30in tables. I don’t know the dimensions of the box but I do know each box holds six 1-gallon plastic water bottles. The boxes are packed with most books laying flat, stacked on top of each other, with a few others standing upright in the remaining space.

My questions are:

1. About how many books can we display spine up on each 8ft x 30in table
2. Approximately how many boxes should I assign to each table for unpacking so I can fill the 8ft x 30in table

Thanks,

The Friends of the Library

In a way it reminds me of MAP’s Money Munchers task I did with my students some time ago. Various book sizes and thicknesses impact the number of books that can be placed in the box, just as various mattress sizes impact the number of dollar bills covering the area in the Money Munchers task.

When giving this task I plan to have on hand an empty 1 gallon container, a Hinckley & Schmidt box, and a sample hardcover book for students who need a visual aid. Rulers will be available should students ask for them.

What can I do to improve this task before I present it to the students? I’d love your thoughts and comments.

The endless chase for the perfect lesson

Sometimes I think we teachers are so invested in our work that we exhaust ourselves and flame out before spring break. What fatigues me the most is the endless chase for the perfect lesson. I feel like I’ve been on a treadmill logging miles, getting tons of exercise, but in the end I’m right back where I started. I can’t tell you how many hours I’ve spent searching 3-Act Tasks, Desmos, Shell, #MTBOS and other resources for that perfect lesson. What caused this endless search and what am I truly looking for?

Shortly after the Common Core was adopted, our math department took on the task of breaking down the standards to write our own curriculum. At the time, you may recall, textbook publishers were simply slapping the Common Core label on their lessons.

Our math department knew there should be something more, but we lacked the time and curriculum development expertise to write a coherent and cohesive scope and sequence. Following a structure and level of rigor based on our previous textbook adoption wasn’t enough. Thus the endless search for the perfect lesson.

Since we’ll be piloting the 6-8 IM curriculum this year, I won’t be searching for the perfect lesson. That’s not to say every IM lesson will be perfect, but we need to stay true to the pilot if we want to evaluate the curriculum with fidelity. This pilot will allow me to hop off that treadmill.

But the question remains, what am I truly looking for in a lesson? If I am to trust that each lesson has clear learning goals and is sequenced appropriately, my job then becomes to ensure continuous, embedded, formative assessment so I can offer effective feedback to advance learning. That’s a mouthful; it may be a cliche; but it’s true.

This year I’ll be spending my time and energy on the least dazzling but most essential part of a lesson.

 

Homework and practice

The 6-8 math teachers received Illustrative Mathematics training this summer and one key question we posted on our “parking lot” is the purpose of homework and daily practice. We will be meeting as a department next week to discuss homework and its role, if at all, in daily instruction. Should we spend class time reviewing each problem? Select problems? Don’t go over it at all? Should we collect and review student homework as a formative assessment tool? Do we scan for a completion grade based on following the criteria for success? Use it as an opportunity to teach responsibility? Do students learn from the worked solutions we provide? Should the practice be blocked or interleaved? There are a myriad of questions we face when evaluating the purpose and effectiveness of homework.

None of the teachers I collaborate with assign “too much” homework. But there’s potential for any homework assignment to slide down the slippery slope from independent practice which can be successfully be completed by the student, to dependent practice involving the parent, tutor, etc. to downright no practice where the student simply copies the worked solutions.

In grades 6-8, students follow a homework criteria for success process of GCS–Grade, Correct, Submit. Grade by marking C, PC or NY; correct each PC or NY using the worked solution, then submit. While a worked solution is a helpful learning tool, I have two issues with using worked solutions: 1) it locks the student into showing work using only one method, and 2) some students simply copy the key and do not use it as a learning tool.

When we have our homework discussion, I would like to hear my colleagues’ opinions on assigning fewer problems from the IM practice, providing worked solutions to similar problems and continuing to have students self correct their work outside of class.

For example if we ask students to complete problem #5 from this grade 6 IM lesson, one worked solution would be provided and the students are then asked to show two other ways.

Here, the advantage is students can reference the worked solution in order to solve it other ways.

This is merely a suggestion to jump start the conversation. And to be sure, this should not be considered a homework policy that every teacher must adhere to. How a teacher uses homework in their instructional decisions is up to them.

#MTBoSBlaugust

 

Tackling Tanton’s Pinwheel Area Problem

While our middle school math summer success program has ended I wanted to share how the students grappled with James Tanton’s Pinwheel Area problem. I was only working with seven students that day because the rest of the class was on an EL field trip.

I gave each student a copy of the problem and 10 minutes of quiet think time before solving as a group.

After 7-8 minutes none of the students were able to get started so I asked, “What shapes do you see?” Their first response was the pinwheel and squares. Then I asked, “What other shapes to you see?” There as a very long pause. I sensed the students eyes were solely focused on the pinwheel and grid so I asked them to look at the entire image. Another long pause.

“Do you see any other shapes besides the pinwheel and squares?”

A few moments passed and a student responded, “I see triangles.”

I should have anticipated this task better. I was expecting to scaffold, but as we moved through the task, the students needed additional support.

“What triangles do you see?” My eyes naturally saw the larger triangles, but his eyes didn’t.  I asked the student to point to the triangles.

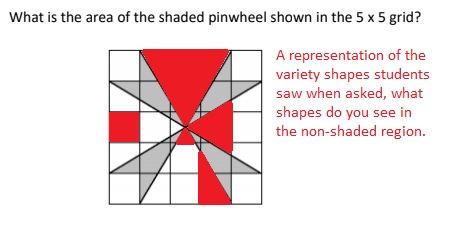
At this point I recognized the grid was a distractor–which is great in terms of adding rigor to the problem while providing another scaffolding opportunity.

Let’s draw this on the board.

 

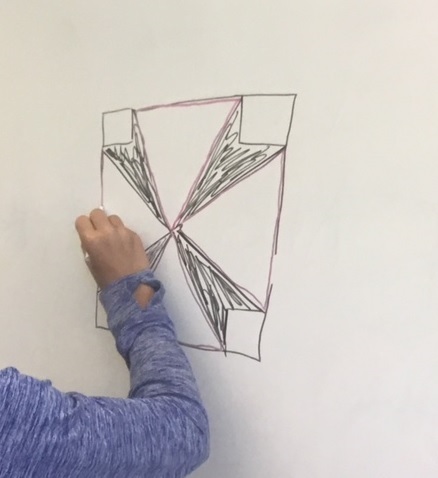
Now we were able to discuss a strategy. It took some time, but we agreed that if we were able to subtract the non-shaded area from the 5×5 grid, we would find the area of the pinwheel.

Students enjoy working on the whiteboard and wanted to shade the triangles.

Students recalled how to find the area of a square or rectangle, but could not remember how to find the area of a triangle. I drew a rectangle, and labeled the length and width as 3×2. “What’s the area?”

“Six.”

I cut the rectangle in half horizontally and asked, “What’s the area of one section?”

“Three.”

I erased the horizontal line and replaced it by cutting the rectangle in half vertically. “What’s the area of this section?”

“Three.”

I erased the vertical line and cut the rectangle in half diagonally. “What’s the area of this section?”

“Three.”

So if we multiply the base and height of a triangle and cut it in half (divide by 2) we can find the area. We revisited the printout of the problem to find the measurements and began calculating.

Now we were able to put it all together.

To achieve productive struggle this group required more scaffolding than I originally anticipated. I need to reread Five Practices.